

To completely describe any parabola, all someone needs to tell you are these three values. Step 2 Move the number term to the right side of the equation: P 2 460P -42000. These three values, a, b, and c, will describe a unique parabola. How would you describe the effect that changing the value of b has on the graph? If you wish to explore this behavior in a bit more depth, you may use this applet. Note what happens to the graph when you set a to a negative value.Ĭ shifts (translates) the graph vertically.ī alters the the graph in a complex way. It determines how much the graph is stretched away from, or compressed towards, the x-axis. Vertex Form: ya (x-h)2+k y a(x h)2 +k Each quadratic form looks unique, allowing for different problems to be more easily solved in one form than another. 1) You can create a table of values: pick a value of 'x' and calculate 'y' to get points and graph the parabola. The 3 Forms of Quadratic Equations There are three commonly-used forms of quadratics: 1. To help with the conversion, we can expand the standard form, and see that it turns into the general form. There are multiple ways that you can graph a quadratic. We know the general form is ax2+bx2+c, and the standard form is a(x-h)2+k.
Standard form of a quadratic how to#
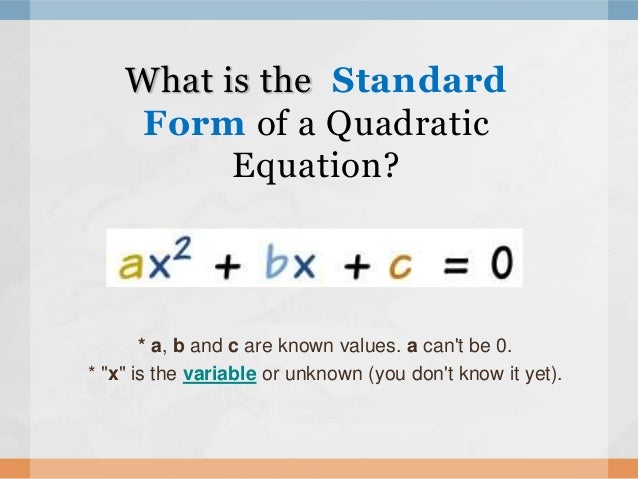
This is much more challenging!Ī is referred to as the "dilation factor". I'm learning how to convert quadratic equations from general form to standard form, in order to make them easier to graph. The graph of a quadratic function is 'U' shaped and is called a parabola. All quadratic equations can be put in standard. Quadratic Functions in Standard Form Quadratic functions in standard form f (x) a (x - h) 2 + k and the properties of their graphs such as vertex and x and y intercepts are explored, interactively, using an applet. the vertex of the graph (the blue point labelled V) passes through the blue point on the graph: (-3, -1). FYI: Different textbooks have different interpretations of the reference standard form of a quadratic function. The standard form of a quadratic equation is ax2 + bx + c 0 when a 0 and a, b, and c are real numbers. some part of the graph passes through the blue point on the graph: (-3, -1) In this form, the quadratic equation is written as.

For example, two standard form quadratic equations are f (x) x 2 + 2x + 1 and f (x) 9x 2 + 10x -8. the graph becomes a horizontal line, or opens down In this form, the quadratic equation is written as: f (x) ax 2 + bx + c where a, b, and c are real numbers and a is not equal to zero. the vertex lies to the right, or left, of the y-axis 4) You can convert the equation into vertex form by completing the square. Once you have a feel for the effect that each slider has, see if you can adjust the sliders so that: 3) If the quadratic is not factorable, you can use the quadratic formula or complete the square to find the roots of the quadratic (the x-intercepts) and then find the vertex as shown in this video.


 0 kommentar(er)
0 kommentar(er)
